Rules and Guidelines

Want to participate in the discussions? Please read our Community Rules and Guidelines. Need some help? Visit Support and Answers.

State Not Answered
Locked Locked
Replies 35 replies
Subscribers 31 subscribers
Views 4643 views
Users 0 members are here

Options

You may also be interested in

This discussion is locked.

You cannot post a reply to this discussion. If you have a question start a new discussion

Appendix 4, section 6.1 equation 6

Former Community Member over 3 years ago

Hi I am working through equation 6 for an ambient temperature of 50 degrees for 1mm 90degC thermoplastic cable (Table 4E2A)

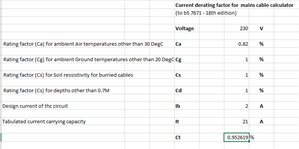
I get a correction factor of 0.95 for a 2A load.

Do i divide the mV/A/m by this factor ?

The text says multiply, but that would mean the resistance decreases with the increase in temperature (or have I got the sum wrong?)

Parents

0 Former Community Member over 3 years ago

Thanks Mike, you two approximations there are both around the 11-12% increase for the effect of temperature, however my 0.95 factor is only half that, from the rules of thumb I would have expected a value closer to 0.88 so it looks like the self-heating of the cable is reducing the effect of the correction factor for ambient in my sum.
In you first post you say “if we changed the Cg, Cs Cd etc are in the direction that they are less than one” - I just took the Ca straight from the table at 0.82 I (assumed this to be a multiplier. Is this actually the % by which the capacity drops?)
I.e should I have flipped that value (1/0.82) to 122% ? If do the sum then works out to 0.89% which is closer to the expected value
This discussion has been great for me, it not about the numerical accuracy of the answer, but understand the principles it is based upon
Cancel
Vote Up 0 Vote Down

Cancel

Reply

0 Former Community Member over 3 years ago

Thanks Mike, you two approximations there are both around the 11-12% increase for the effect of temperature, however my 0.95 factor is only half that, from the rules of thumb I would have expected a value closer to 0.88 so it looks like the self-heating of the cable is reducing the effect of the correction factor for ambient in my sum.
In you first post you say “if we changed the Cg, Cs Cd etc are in the direction that they are less than one” - I just took the Ca straight from the table at 0.82 I (assumed this to be a multiplier. Is this actually the % by which the capacity drops?)
I.e should I have flipped that value (1/0.82) to 122% ? If do the sum then works out to 0.89% which is closer to the expected value
This discussion has been great for me, it not about the numerical accuracy of the answer, but understand the principles it is based upon
Cancel
Vote Up 0 Vote Down

Cancel

Children

No Data

Join us to get the best from IET EngX.

Joining EngX lets you personalise your experience so you stay up to date on the topics that interest you, plus you’ll be able to make connections who are looking to collaborate, exchange ideas and more.