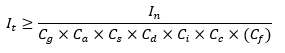Im getting a bit confused with It and Iz, and which is out final value when calculating cable size
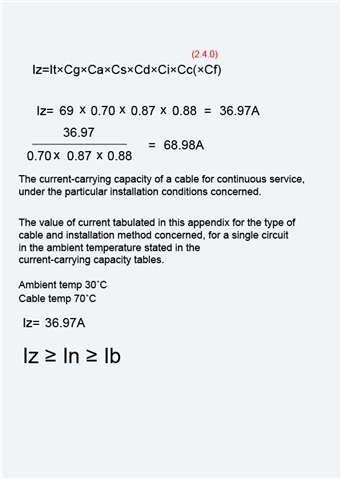
It - the value of current tabulated in this appendix for the type of cable and installation method concerned, for a single circuit in the ambient temperature stated in the current-carrying capacity tables
Iz - the current-carrying capacity of a cable for continuous service, under the particular installation conditions concerned.
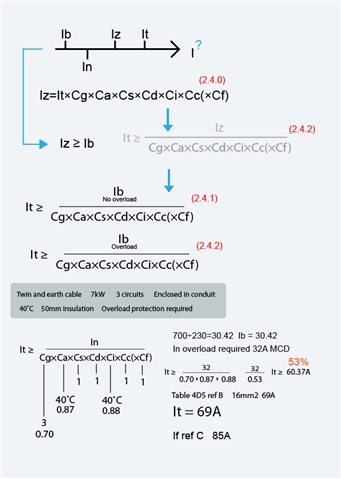
In =32 Ca 0.87
so It ≥ 32 / 0.87
It ≥ 36.78A
Look up tables in BS 7671 clipped direct (c) one 2 core cable
4mm=36A
6mm= 46A
So we go with 46A
So our It (Tabulated value we look up) is 46A and we will use a 6mm2 cable
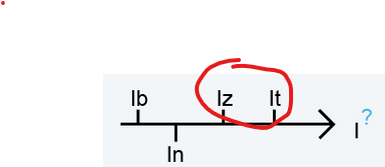
So what is Iz?
Iz ≥ In ≥ Ib understand the relationship
Now Iz = It x correction factors.
Iz = 46 x 0.87 = 40.02A which is greater than In (32A) so thats OK
But we got a value of 36.78 A when applying correction factors to In
We also have this formula...
It ≥ Iz / correction factors
40.02 / 0.87 = 46
We end up with the same number.
We have a calculated value of 36.78A in the book the cable choice is 6mm which is rated at 46A
I'm struggling to see what we would call our final cable choice? Its not Iz , but the description (continuous service) makes it sound like it is
What is Iz
Hope that make some sense
Its based around this IET article