This discussion is locked.

You cannot post a reply to this discussion. If you have a question start a new discussion

Max Zs BS7671 17th Edition / Guidance Note 3 Inspection and Testing

Former Community Member over 5 years ago

Hello,

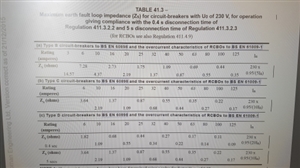
There seems to be some slight differences with regards to acceptable Zs values when comparing the two documents BS7671 17th Edition A3 and Guidance Note 3 Inspection and Testing 17th Edition A3.

For example table 41.3 from BS7671 17th Edition A3 states:

0.4sec trip D6A = 1.82 Ω

Adjusted to 80% as per appendix 14 = 1.456 Ω

However page 121 from Guidance Note 3 Inspection and Testing 17th Edition A3 states:

0.4sec trip D6A = 1.46 Ω

These two documents are produced by IET. Guidance Note 3 Inspection and Testing 17th Edition A3 is rounding up Zs values above the maximum allowable values detailed in BS7671 17th Edition A3, why is that ?

ba51c8f3cf43a4eb3610fe4e2008860e-huge-20190722_083125.jpg

5d6415d8285e515a6e1d04f5ef5fb4b8-huge-20190722_083103.jpg

0 Former Community Member over 5 years ago

@mapj1

Issue = IET contradictions in official documentations regards maximum allowable Zs values.

measure Zs on a real system = irrelevant to the above mentioned issue.

measure values at different times of day = irrelevant to the above mentioned issue.

meter accuracy = irrelevant to the above mentioned issue.

meter lead resistance = irrelevant to the above mentioned issue.

typical spec = irrelevant to the above mentioned issue.

The contradiction may be deemed small and negligible and be explained away as such but at the end of the day it is still a contradiction.

If the IET issue a document defining a value as the the maximum then they should not issue another document contradicting said document.
Cancel
Vote Up 0 Vote Down

Cancel
0 mapj1 over 5 years ago

They can be as contrary as they like I'm afraid, and they are, on many matters far more weighty than this one of varying precision.

It's only paper. The real world is engineered.
Cancel
Vote Up 0 Vote Down

Cancel
0 Legh Richardson over 5 years ago

Lol ....

'' The problem in "engineering" terms or any other terms for that matter is 1.4566666667 Ω does not equal 1.46 Ω. ''

There were a couple of Russians, approx 30 years ago, living in a New York flat working on the number of decimal places without repitition of Pi. They got to 5,000,000 dps !

How they managed to get their mainframe up and running in a block of flats defies logic and utility bills !

Legh...
Cancel
Vote Up 0 Vote Down

Cancel
0 AJJewsbury over 5 years ago

The problem in "engineering" terms or any other terms for that matter is 1.4566666667 Ω does not equal 1.46 Ω.

:

In any case I have not heard a valid reason as to why the IET would round up Zs values above the maximum allowed by BS7671 instead of rounding them down if they want to display them to 2 decimal places.

OK, imagine (just theoretically) you had a circuit where max Zs precisely equalled 1.4560 Ohms - is that a pass or a fail situation?

You then measured the loop impedance with a 100% accurate loop meter that could display to 2 d.p. What would you expect the meter to display? What would you conclude from that reading?

(You might also like to research how the 80% figure comes about (clue: 0.004 per degree C) and what effect actual conditions during measurement might have on results.)

- Andy.
Cancel
Vote Up 0 Vote Down

Cancel
0 AJJewsbury over 5 years ago

There were a couple of Russians, approx 30 years ago, living in a New York flat working on the number of decimal places without repitition of Pi. They got to 5,000,000 dps !

How they managed to get their mainframe up and running in a block of flats defies logic and utility bills !

And (supposedly) the Americans got to the moon by using just 6 decimal places for pi!

- Andy.
Cancel
Vote Up 0 Vote Down

Cancel
0 Legh Richardson over 5 years ago

AJJewsbury:

There were a couple of Russians, approx 30 years ago, living in a New York flat working on the number of decimal places without repitition of Pi. They got to 5,000,000 dps !

How they managed to get their mainframe up and running in a block of flats defies logic and utility bills !

And (supposedly) the Americans got to the moon by using just 6 decimal places for pi!

- Andy.

I'm not sure whether they could have got any greater accuracy as the processing power of the time was something akin to 4 16-bit registers with 11-bit memory capability...

Apparantly, Pi dps are very useful for encrytion purposes

Legh
Cancel
Vote Up 0 Vote Down

Cancel
0 AJJewsbury over 5 years ago

I'm not sure whether they could have got any greater accuracy as the processing power of the time was something akin to 4 16-bit registers with 11-bit memory capability...

You're not limited to the machine's word-size - you can calculate and store across several adjacent memory locations using the equivalent of 'long' calculations (e.g. do one byte/word at a time and carry/borrow from one to the next). The old 6502 based systems I used to work with (many years ago) supported calculations (IIRC) between 1E38 and 1E-38 with 6 significant decimal digits even though the hardware registers and memory were all just 8-bit (-128 to 127 integers only).

- Andy.
Cancel
Vote Up 0 Vote Down

Cancel
0 Chris Pearson over 5 years ago

Mike M:
Chris Pearson‍

That's very presumptuous of you considering you don't know what meters I have used.

Once again taking into account all that we know about tolerances and circuit variations, if something is defined as the maximum then surely it should it should never be acceptable to have a value higher than the maximum as far as the regs are concerned.

Please don't make the mistake of thinking that just because a meter displays 2 dp, it is accurate to 2 dp.

If maximum really must be a maximum, it follows that a meter must never under-read. This is rather like car speedos. In reality the meter might, within 99% confidence, measure on a 0 - 1 Ω scale +/- 0.05 Ω, so if it gives a reading of 1.00 Ω, 95% of the time, the true value will be between 0.95 Ω and 1.05 Ω.

If the true value must never be under-estimated, what we now do is oblige the meter to be accurate to within +0.1 Ω/-0.0 Ω. That is achieved by making the meter over-read, on average, by 0.05 Ω.

So in pursuit of making maximum = maximum, you have made the meter less accurate.

And you still have a 0.5% chance of under-reading.
Cancel
Vote Up 0 Vote Down

Cancel

Max Zs BS7671 17th Edition / Guidance Note 3 Inspection and Testing

Join us to get the best from IET EngX.

Community Rules & Guidelines