In order to size a load carrying conductor after a circuit breaker, I've historically included an I2t calculation to ensure the cable used has a sufficient cross-sectional area in the event of a fault, to ensure the insulation doesn't damage.
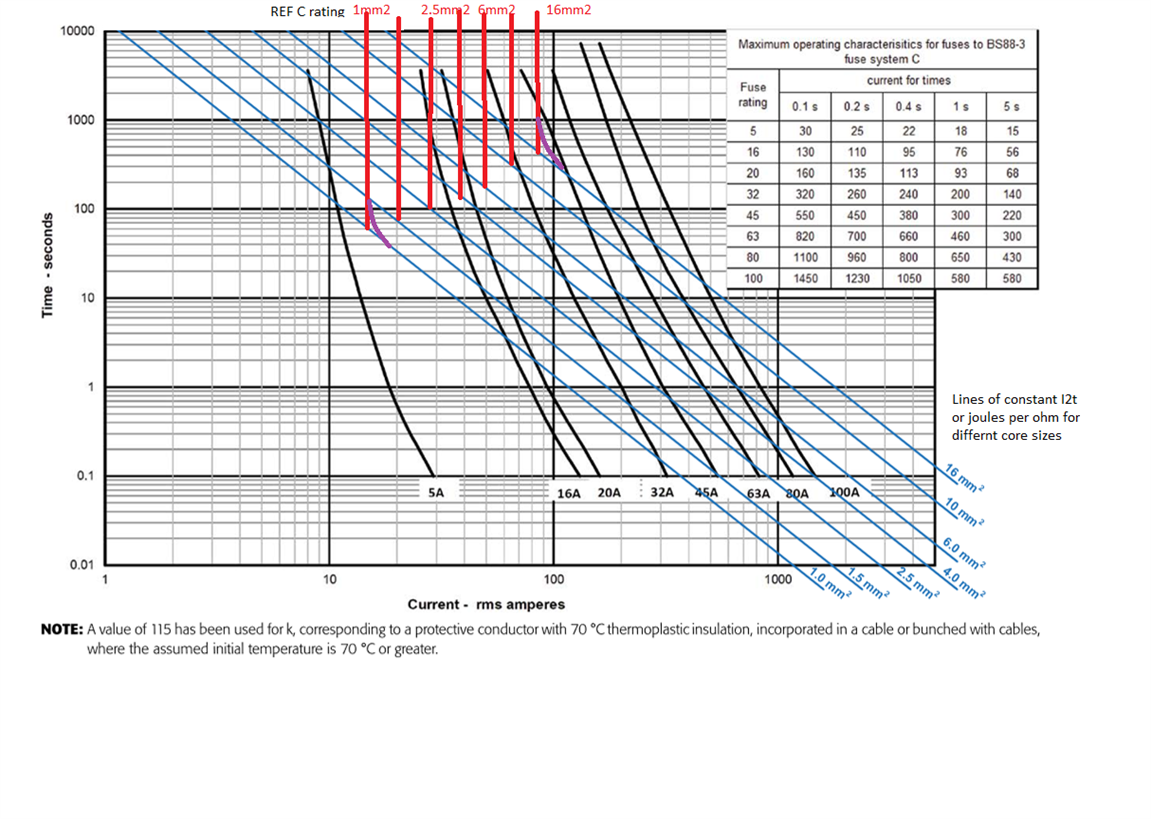
To do this, I use the Time-Current Curve available for the breaker to calculate the worst case A2s. ( Fault Current^2 (A) x Trip Time (s) )
The Time Current curve for the part model I use shows the curve flattening at 1000s - this has historically been where I've calculated the worst case A2s.
I've recently been reading on the subject and talking to other Engineers, and I've been told that for trip times beyond 5s, the Adiabatic equation isn't really applicable.
Beyond that, if you plot a bell curve of Fault Duration against its likelihood to occur, then using a boundary of 5s would likely cover up to the 95th percentile of possible faults - which offers a good balance between safety, reliability and cost.
If the adiabatic equation is no longer applicable beyond 5s, then I obviously need to find a more appropriate way to calculate it - however I'm not convinced that a 5s boundary would cover the 95th percentile of possible faults.
Is the reasoning sound here? If so, does anyone know where this is possibly stated, to offer reasoning behind the decision?
If it is true; the 95-100th percentile of faults could cause a fire in the absolute worst case scenario, so how do you quantify the risks with that?
If not, should I be calculating up to the boundaries that the manufacturer tested for (i.e. 1000s), or is there a better argument for a lower value such as 5s?
If I've misunderstood the subject here, your help would be appreciated.