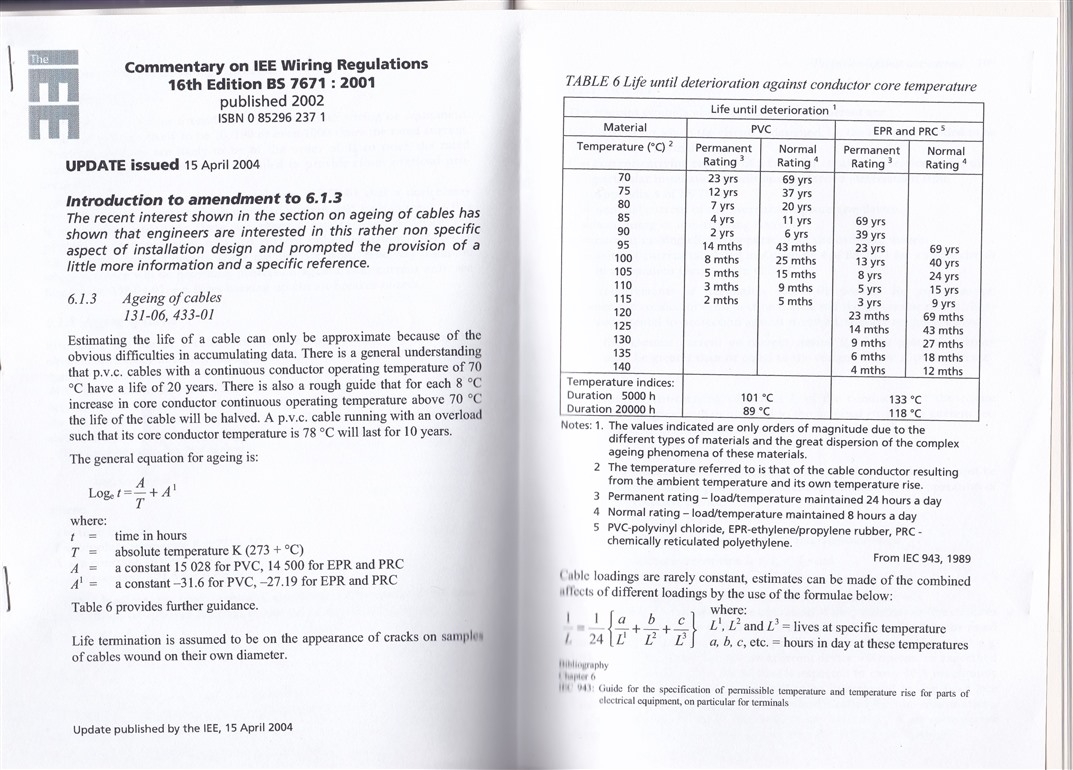
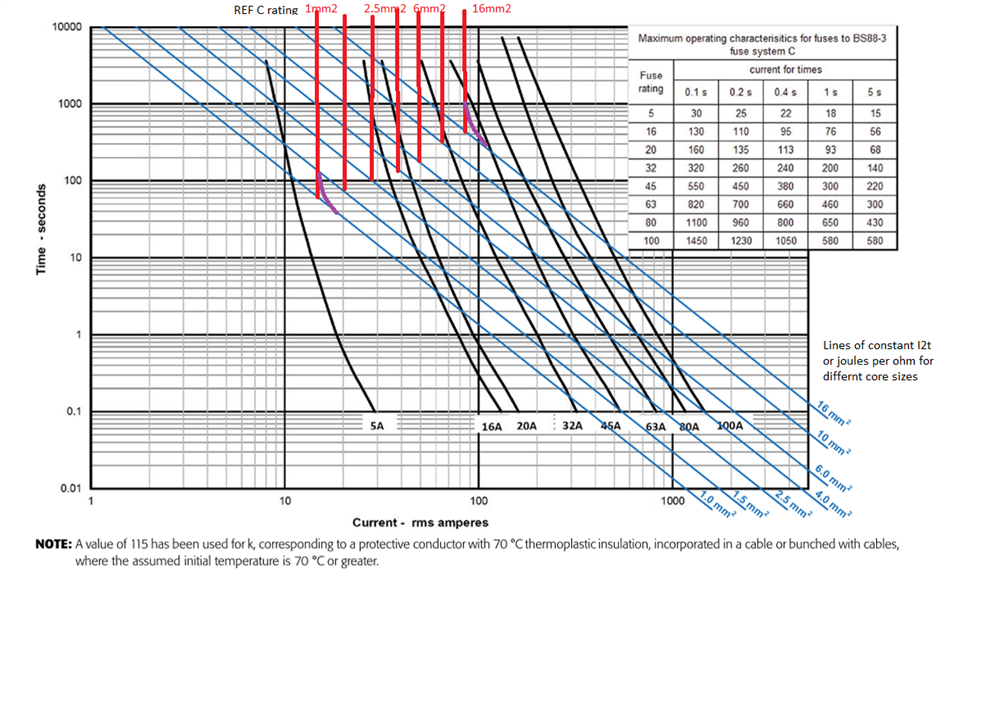
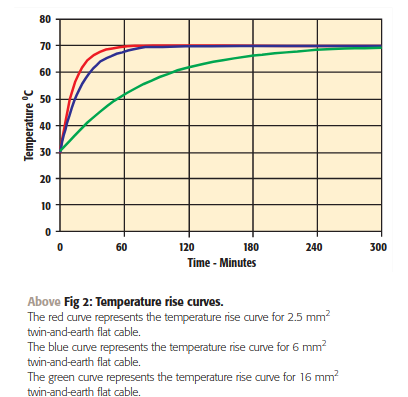
When using adiabatic equation for calculating minimum size of CPC, every example I have seen uses 0.1 second or whatever the disconnect time of the mcb element of the RCBO or MCB will be.
In a domestic sittuation most circuits are protected by RCD's with a trip time of 40mS with significant fault currents, in this sittuation why don't we use 40mS as T in the adiabatic equation?